Alireza Shamkhalchian devoted his PhD on the computational simulation of (in particular high-scale) flood inundation problems, where the simulations are a powerful tool for the risk mapping of floods as a central part of decision-making in the field of environmental management.
The motivation of his research can be described as follows. Over the past decades giant achievements have been attained in computational hydraulics and a number of accurate models have emerged. However, the accurate simulations of these models require high-resolution meshes, resulting in high computational cost. Thus, the models providing a rational trade-off between accuracy and time cost are highly demanded. His PhD is defined in the light of that where the lack of such tools substantially limits our ability to understand and predict floods that extend over large areas.
The model solves the two-dimensional shallow water equations (SWE) by a Godunov type finite volume (FV) method that makes use of two nested meshes as shown in the figure. Runtime computations are performed at a coarse computational mesh (thus increasing computational performance), while a fine mesh is used to incorporate finely resolved information into the solution at pre-processing level (thus substantially reducing the loss of accuracy that would otherwise result). The proposed model presents new upscaling methods that are separately derived for each of the terms in the SWE based on the integration of the governing equations over subdomains defined by the coarse resolution grid cells, such as friction and bed slope source terms. This research also sheds light onto the limitations of some of the widely used assumptions in nested meshes models, such as constant friction slope for upscaling the friction effects.
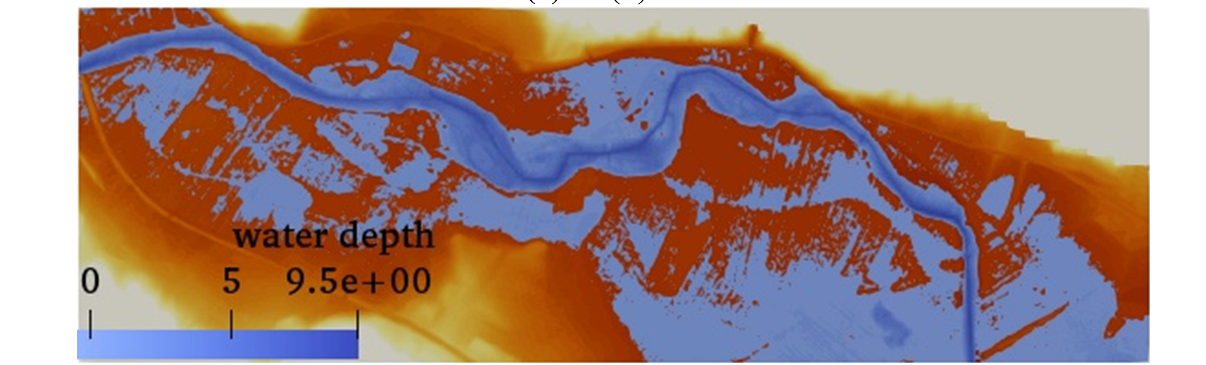
The developed model was tested against a number of real world and artificial test cases including those shown in the figures. It is shown that that i) in general the proposed model provides more accurate results than traditional FV models currently available and ii) for the same accuracy and at low resolution, the proposed methods substantially increase computational performance.
Recently computational models have gained many applications in flood inundation problems, so that in the engineering marketing many models compete with others. This is the reason that Jacobs Engineering Group, which is the owner of the commercial product FloodModeller, sponsored this study. A copy of the model developed at the University of Southampton has been delivered to Jacobs Engineering Group for commercial purposes.
References:
Shamkhalchian, A., de Almeida, G. A. (2020). Upscaling the shallow water equations for fast large scale flood modelling. Journal of Hydraulic research, https://doi.org/10.1080/00221686.2020.1818316.